Last Sunday the eminent British historian of the twentieth century, Richard Evans, tweeted the following:
Let’s remember we use Arabic numerals – 1, 2, 3 etc. Try dividing MCMLXVI by XXXIX – Sir Richard Evans (@Richard Evans36)
There was no context to the tweet, a reply or whatever, so I can only assume that he was offering a defence of Islamic or Muslim culture against the widespread current attacks by drawing attention to the fact that we appropriated our number system along with much else from that culture. I would point out, as I have already done in my nineteenth-century style over long title, that one should call them Hindu-Arabic numerals, as although we appropriated them from the Islamic Empire, they in turn had appropriated them from the Indians, who created them.
As the title suggests, in his tweet Evans is actually guilty of perpetuating a widespread and very persistent myth concerning the comparative utility of the Hindu-Arabic number system and the Roman one when carrying out basic arithmetical calculations. Although I have taken Professor Evans’ tweet as incentive to write this post, I have thought about doing so on many occasions in the past when reading numerous similar comments. Before proving Professor Evans wrong I will make some general comments about the various types of number system that have been used historically.
Our Hindu-Arabic number system is a place-value decimal number system, which means that the numerals used take on different values depending on their position within a given number if I write the Number of the Beast, 666, the three sixes each represents a different value. The six on the far right stands for six times one, i.e. six, its immediate neighbour on the left stands for six time ten, i.e. sixty, and the six on the left stands for six times one hundred, i.e. six hundred, so our whole number is six hundred and sixty six. It is a decimal (i.e. ten) system going from right to left the first numeral is a multiple of 100 (for those who maths is a little rusty, anything to the power of zero is one), the second numeral is a multiple of 101, the third is a multiple of 102, the forth is a multiple of 103, the fifth is a multiple of 104, and so on and so fourth. If we have a decimal point the first numeral to the right of it is 10-1 (i.e. one tenth), the second 10-2 (i.e. one hundredth), the third 10-3 (i.e. one thousandth), and so on and so forth. This is a very powerful system of writing numbers because it comes out with just ten numerals, one to nine and zero making it very economical to write.
The Hindu-Arabic number system developed sometime in the early centuries CE and our first written account of it is from the Indian mathematician, Brahmagupta, in his Brāhmasphuṭasiddhānta (“Correctly Established Doctrine of Brahma“) written c. 628 CE. It came into Europe via Al-Khwārizmī’s treatise, On the Calculation with Hindu Numerals from 825 CE, which only survives in the 12th-century Latin translation, Algoritmi de numero Indorum. After its initial introduction into Europe in the high Middle Ages the Hindu-Arabic system was only really used on the universities to carry out computos, that is the calculation of the dates on which Easter falls. Various medieval scholars such as Robert Grosseteste John of Sacrobosco wrote elementary textbooks explaining the Hindu-Arabic system and how to use it. The system was reintroduced for trading purposes by Leonard of Pisa, who had learnt it trading with Arabs in Spain, in his book the Liber Abbaci in the thirteenth century but didn’t really take off until the introduction of double-entry bookkeeping in the fourteenth century.
The Hindu-Arabic system was not the earliest place-value number system. That honour goes to the Babylonians, who developed a place-value system about 1700 2100* BCE but was not a decimal system but a sexagesimal system, that is base sixty, so the first numeral is a multiple of 600, the second a multiple of 601, the third a multiple of 602, and so on and so fourth. Fractions work the same, sixtieths, three thousand six-hundredths (!), and so on and so fourth. Mathematically a base sixty system is in some senses superior to a base ten one. The Babylonian system suffered from the problem that it did not have distinct numerals but a stroke list system with two symbols, one for individual stroke and a second one for ten stokes:
The Babylonian system also initially suffered from the fact that it possessed no zero. This meant that to take the simple case, apart from context there was no way of knowing if a single stroke stood for one, sixty, three thousand six hundred or whatever. The problem gets even more difficult for more complex numbers. Later the Babylonians developed a symbol for zero. However the Babylonian zero was just a placeholder and not a number as in the Hindu-Arabic system.
The Babylonian sexagesimal system is the reason why we have sixty minutes in an hour, sixty seconds in a minute, sixty minutes in a degree and so forth. It is not however, contrary to a widespread belief the reason for the three hundred and sixty degrees in a circle; this comes from the Egyptian solar years of twelve thirty day months projected on to the ecliptic, a division that the Babylonian then took over from the Egyptians.
The Greeks used letters for numbers. For this purpose the Greek alphabet was extended to twenty-seven letters. The first nine letters represented the numbers one to nine, the next nine the multiples of ten from ten to ninety and the last nine the hundreds from one hundred to nine hundred. For the thousands they started again with alpha, beta etc. but with a superscript prime mark like an apostrophe. So twice through the alphabet takes you to nine hundred thousand nine hundred and ninety-nine. If you need to go further you start at the beginning again with two primes. Interestingly the Greek astronomers continued to use the Babylonian sexagesimal system, a tradition in the astronomy that continued in Europe down to the Renaissance.
We now turn to the Romans, who also have a simple stroke number system with a cancelled stroke forming an X as a bundle of ten strokes. The X halved horizontally through the middle gives a V for a bundle of five. As should be well known L stands for a bundle of fifty, C for a bundle of one hundred and M for a bundle of one thousand given us the well known Roman numerals. A lower symbol placed before a higher one reduces it by one, so LX is sixty but XL is forty. Of interest is the well-known IV instead of IIII for four was first introduced in the Middle Ages. The year of my birth 1951 becomes in Roman numerals MCMLI.
When compared with the Hindu-Arabic number system the Greek and Roman systems seem to be cumbersome and the implied sneer in Professor Evans’ tweet seems justified. However there are two important points that have to be taken into consideration before forming a judgement about the relative merits of the systems. Firstly up till the Early Modern period almost all arithmetic was carried out using a counting-board or abacus, which with its columns for the counters is basically a physical representation of a place value number system.
The oldest surviving counting board dates back to about 300 BCE and they were still in use in the seventeenth century.
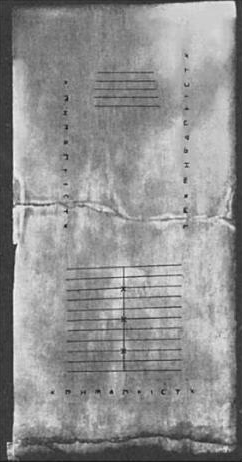
An early photograph of the Salamis Tablet, 1899. The original is marble and is held by the National Museum of Epigraphy, in Athens.
Source: Wikimedia Commons
A skilful counting-board operator can not only add and subtract but can also multiply and divide and even extract square roots using his board so he has no need to do written calculation. He just needed to record the final results. The Romans even had a small hand abacus or as we would say a pocket calculator. The words to calculate, calculus and calculator all come from the Latin calculi, which were the small pebbles used as counters on the counting board. In antiquity it was also common practice to create a counting-board in a sand tray by simply making parallel groves in the sand with ones fingers.

A reconstruction of a Roman hand abacus, made by the RGZ Museum in Mainz, 1977. The original is bronze and is held by the Bibliothèque nationale de France, in Paris. This example is, confusingly, missing many counter beads.
Source: Wikimedia Commons
Moving away from the counting-board to written calculations it would at first appear that Professor Evans is correct and that multiplication and division are both much simpler with our Hindu-Arabic number system than with the Roman one but this is because we are guilty of presentism. In order to do long multiplication or long division we use algorithms that most of us spent a long time learning, often rather painfully, in primary school and we assume that one would use the same algorithms to carry out the same tasks with Roman numerals, one wouldn’t. The algorithms that we use are by no means the only ones for use with the Hindu-Arabic number system and I wrote a blog post long ago explaining one that was in use in the early modern period. The post also contains links to the original post at Ptak Science books that provoked my post and to a blog with lots of different arithmetical algorithms. My friend Pat Belew also has an old blog post on the topic.
I’m now going to give a couple of simple examples of long multiplication and long division both in the Hindu-Arabic number system using algorithms I learnt I school and them the same examples using the correct algorithms for Roman numerals. You might be surprised at which is actually easier.
My example is 125×37
125
37
875 Here we have multiplied the top row by 7
3750 Here we have multiplied the top row by 3 and 10
4625 We now add our two partial results together to obtain our final result.
To carry out this multiplication we need to know our times table up to nine times nine.
Now we divide 4625 : 125
4625 : 125 = 37
375
875
875
000
First we guestimate how often 125 goes into 462 and guess three times and write down our three. We then multiply 125 by three and subtract the result from 462 giving us 87. We then “bring down” the 5 giving us 875 and once again guestimate how oft 125 goes into this, we guess seven times, write down our seven, multiply 125 by 7 and subtract the result from our 875 leaving zero. Thus our answer is, as we already knew 37. Not exactly the simplest process in the world.
How do we do the same with CXXV times XXXVII? The algorithm we use comes from the Papyrus Rhind an ancient Egyptian maths textbook dating from around 1650 BCE and is now known as halving and doubling because that is literally all one does. The Egyptian number system is basically the same as the Roman one, strokes and bundles, with different symbols. We set up our numbers in two columns. The left hand number is continually halved down to one, simple ignoring remainders of one and the right hand is continually doubled.
| 1 | XXXVII | CXXV |
| 2 | XVIII | CCXXXXVV=CCL |
| 3 | VIIII | CCCCLL=CCCCC=D |
| 4 | IIII | DD=M |
| 5 | II | MM |
| 6 | I | MMMM |
You now add the results from the right hand column leaving out those were the number on the left is even i.e. rows 2, 4 and 5. So we have CXXV + D + MMMM = MMMMDCXXV. All we need to carry out the multiplication is the ability to multiply and divide by two! Somewhat simpler than the same operation in the Hindu-Arabic number system!
Division works by an analogous algorithm. So now to divide 4625 by 125 or MMMMDCXXV by CXXV
| 1 | I | CXXV |
| 2 | II | CCXXXXVV=CCL |
| 3 | IIII | CCCCLL=CCCCC=D |
| 4 | IIIIIIII=VIII | DD=M |
| 5 | VVIIIIII=XVI | MM |
| 6 | XXVVII=XXXII | MMMM |
We start with 1 on the left and 125 on the right and keep doubling both until we reach a number on the right that when doubled would be greater than MMMMDCXXV. We then add up those numbers on the left whose sum on the right equal MMMMDCXXV, i.e. rows 1, 3 and 6, giving us I+IIII+XXXII = XXXIIIIIII = XXXVII or 37.
Having explained the method we will now approach Professor Evan’s challenge
| 1 | I | XXXIX=XXXVIIII |
| 2 | II | XXXXXXVVIIIIIIII=LXXVIII |
| 3 | IIII | LLXXXXVVIIIIII=CLVI |
| 4 | IIIIIIII=VIII | CCLLVVII=CCCXII |
| 5 | VVIIIIII=XVI | CCCCCCXXII=DCXXIIII |
| 6 | XXVVII=XXXII | DDCCXXXXIIIIIIII=MCCXLVIII |
Adding rows 6, 5 and 2 on the right we get MCCXLVIII+CLVI+LXXVIII=MCML i.e. MCMLXVI less XVI so our result is XXXII+XVI+II = L remainder XVI. Now that wasn’t that hard was it?
Interestingly the ancient Egyptian halving and doubling algorithms for multiplication and division are, in somewhat modified form, how modern computers carry out these arithmetical operations.
* Added 13 February 2017: I have been criticised on Twitter, certainly correctly, by Eleanor Robson, a leading expert on Cuneiform mathematics, for what she calls a sloppy and outdated account of the sexagesimal number system. For those who would like a more up to date and anything but sloppy account then I suggest they read Eleanor Robson’s (not cheap) Mathematics in Ancient Iraq: A Social History, Princeton University Press, 2008

